Conjugate Projections
The conjugate projections (180° apart from each other) in SPECT imaging are not the same.
Reasons of this include the fact that the detector (collimator) response is depth-dependent, and the fact that the gamma photons are scattered in the body and the photoelectric effect may occur. Despite this, it can be proven that the conjugate projections carry redundant information. The proof can be understood with the potato-peeler perspective, which uses the fact that the object to be imaged has a convex envelope.
In accordance with that, if the gamma ray attenuation and the detector-collimator response is known and can be well modelled, it is sufficient to take a 180° image. However, it was proven that the angle range in which the 180° image is composed does matter. The more continuous the solid angle in which the projections are formed is, the worse the signal-to-noise ratio of the image will be. This is shown in the figures below, where the upper part depicts the angle ranges in which the images were taken, and the achievable results of reconstruction are visible under them.
As mentioned above, we can make use of the redundant projection if we know the distance-dependent imaging of the collimator. To model this, there exists an analytical solution called frequency-distance principle (FDP). Thus, the frequency components of the points at a given D+l distance from the collimator are aligned around a straight line in the two-dimensional Fourier space of the sinogram, moreover, around a straight line the slope of which equals -l.
This makes it possible to design an inverse filter, this way sharpening the image:
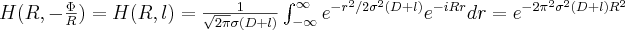
where the standard deviation of the Gaussian filter (H) is dependent on the (D+l) distance: 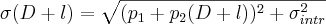
The only problem with this is that sharpening always means the amplification of noise as well. That is why the sharpening filter has to be combined with an adequate (adaptive) low-pass filter or filtering technique that will work against the sharpening. When adjusting the two filters, the fact that the resolution of the projection image is determined by the signal-to-noise ratio needs to be taken into consideration.
The advantage of FDP filtering would be the possibility of reconstructing the image it filtered by anything; however, this is not entirely the case. The reason of this is that after filtering the image statistics does not follow the Poisson distribution, on which the ML-EM reconstruction is based. This problem is illustrated by the series of images below, in which the reconstruction of a simulated Derenzo phantom is depicted (quasi-noise-free: left column, noisy: right column). In the first line the reconstruction algorithm is purely EM, in the second line it is Wiener-filtered FDP+EM while in the third line it is wavelet-filtered FDP+EM.
The other requirement to be met when applying the potato-peeler perspective is to know the gamma-ray attenuation of the medium. Knowing the attenuation, corrections may be performed (AC correction), which are also meant to restore the decrease, the ‘loss’ of activity arriving from the centre of the object.
In order to achieve this, we can start off with the Beer-Lambert law, which describes both scattering and absorption. However, the exponential Radon transform obtained this way has an analytical solution only in one case: in a homogeneous convex absorbing medium, so its implementation is easier in ML-EM reconstruction than in filtered backprojection. Attenuation can be built in the system matrix at the cost of it increasing the computational requirements, but by using it even the posterior wall of the heart can be imaged, the activity of which would otherwise be lost. The reconstruction results of ring-shaped phantoms in an inhomogeneous medium are different in case of a reconstruction containing only OSEM (left side) and a reconstruction containing collimator and attenuation modelling OSEM (right side):
Nonetheless, the Beer-Lambert law is not suitable for creating an entirely accurate attenuation correction, since it describes a different arrangement from what is realized in gamma cameras due to the presence of the collimator. The actual attenuation in the exponent is not linear and is dependent on the energy window. It can be proven by Monte Carlo simulation that following the Beer-Lambert law causes an error of ~5%.
The medium also causes smearing as well as attenuation in the image because of the scattering, which needs to be taken into consideration, since already at an energy as low as 140 keV the scattering dominates over the photoelectric effect. Scatter correction is possible in several ways.
- Deconvolution in the projection image. Its disadvantage is that it amplifies noise.
- In case of collecting data in two or more energy windows the scatter-corrected image will be a linear combination of the images collected in the different energy windows. The accurate proportions can be optimized with measurements. Its disadvantage is that it spoils the Poisson statistics.
- Integrating the modelling of the scattering process in the reconstruction (e.g. by Monte Carlo transport models). Its disadvantage is that it increases computational time.
The conjugate projections still remain redundant even after integrating the modelling of the scatter and the detector response into the reconstruction and the dependence of the signal-to-noise ratio on the sampled angle range also remains. This dependence sets new challenges for developers, since the question arises as to how the projection with the best signal-to-noise ratio can be chosen for organs with known absorption maps before taking the image. In this case only as many projections need to be taken as are sufficient to reconstruct the organ while keeping all the interesting diagnostic information. This new direction of imaging is called adaptive imaging.
::