Determination of heart’s heamodynamic parameters by first pass procedure

Book for Physicists » Fundaments of Digital Image Registration and Image Post-Processing » Dynamic examinations » Determination of heart’s heamodynamic parameters by first pass procedure

By means of the below described method with a single well injected bolus pharmaceutical it is possible to estimate the cardiac output and the systemic circulation’s blood flow parameters of the heart in non-invasive and reproducible way. The acquisition method as well as the technical conditions of the radiopharmaceutical injection is completely the same as were previously at the 4.3.2 chapter. Major difference is in the evaluation procedure. Since the investigated patients may have distinct gender, various weight, height and circulating blood volume therefore it is necessary to normalize some of the calculated parameters by the patient’s body surface for more reliable and more accurate comparisons as well as for better following up. Body weight BW[kg] and body height BH[kg] of the patients can be determined by measurement procedures, while the body surface may be estimated by special nomogram and/or empirical formula (Du Bois). The circulating blood volume can be estimated by nomogram and/or empirical formula (Du Bois) as well as is possible to measure by RIA method. The empirical formula is gender dependent. It is need to remark both the body surface and the circulating blood volume empirical formula are valid for average developed adults (i.e. at least older then 16years). In case of children and less developed teens (16-18years old) is better to use the nomogram for both body surface and circulating blood volume estimation (nomogram provide more reliable value in these particular cases). The adults’ body surface and circulating blood volume empirical formulas are described in the followings:
Empirical body surface BS[m^2] estimation:

$BS[m^2] = 0.007184 * BW^{0.425} * BH ^{0.725}$ (Du Bois method) (2)

Circulating blood volumen BV[lit.] or BV[dm^3] empirical formulas (Du Bois):

In case of males: $BV_{Male}[lit.] = 0.0248 * BH^{0.725}* BW^{0.423} -1.954$

In case of females: $BV_{Female}[lit.] = 0.0236 * BH^{0.725}* BW^{0.423}-1.229$ (Du Bois method)

Let’s consider the figures below to determination and interpret of the flowing parameters:

Figure 20. Frame sequence of first pass hear (frame selection)	Figure 21. Right ventricle ROI definition by frame sequence
Figure 22. Left ventricle ROI definition by frame sequence	Figure 23. Common presentation of the left and right ventricle ROIs for optional background (BG) ROI drawing

There are curves belonging to the defined ROIs and based on their analysis it is possible to obtain the flowing parameters regarding to the heart - heamodynamic parameters -. Figure 24. below shows the so-called theoretical first pass curves of the ventricles to be very similar shape to the real measured curves.

Figure 24. First pass curves of the heart with the curve points and curve domains to be marked for the evaluation.

Cardiac output CO[lit./min] is calculated by the following formula: [2] [4] [5]

CO[lit./min] = 0.85*60[1/min] *BV[lit.] *CST[counts] / A[counts]

The main meaning of the above definition is the circulated blood volume during 1min. The CST[counts] and A[counts] represents the constant radiopharmaceutical distribution, ie. constant activity in the heart volume due to the multiple recirculition flowing process (CST’s are obtained by constant fitting in [t_k;t_l] interval respectively to the 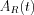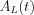A_R(t) , A_L(t) , activity curves of the ventricles). Interpretation of parameter A is more complex. Theoretically A represents the area under the „purely” first pass process of the measured curve, i.e. the total counts of the pure first pass. Let suppose, the beginning of the descending part of the first pass curve can be modelled as an exponential process. Consequently, the complete „pure” first pass process consists of two part: one is the real measured part (ascendant part of the curve till the max.) and the descending part with the exponential fitted curve. Let’s see all these formulas:
Right ventricle first pass curve: $$ A_{Right} = \int\limits_{0}^{\infty} A_R(t)dt = \int\limits_{0}^{ T_{Right Max}} A_R(t)dt + \int\limits_{T_{Right Max}}^{\infty} C_0 e^{-\sigma t}dt$
Left ventricle first pass curve: 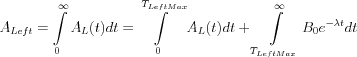$$ A_{Left} = \int\limits_{0}^{\infty} A_L(t)dt = \int\limits_{0}^{ T_{Left Max}} A_L(t)dt + \int\limits_{T_{Left Max}}^{\infty} B_0 e^{-\lambda t}dt$
Thus the cardiac output for both left ventricle and right ventricle can be calculated. Behind the cardiac output the body surface normalized so-called cardiac output index can be derived as follow:
COI[lit./m^2*min] = CO/BS
Then further flowing parameters are determined as well.
Stroke volume: SV[ml] = 1000*CO/HR , where (Heart Rate) is the number heart beats by minute.

Stroke volume index is the SV stroke volume normalized by body surface:

Finally, let us determine the Mean Pulmonary Transit Time for pulmonary circulation being the best estimable and reproducible parameters from the heamodynamic parameters:
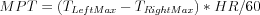$MPT=(T_{LeftMax} - T_{RightMax})*HR/60$ , where the obtained value will present, averagely how many heart cycle is necessary for a complete lung circulation.

Figures below show by pictures how it is possible to obtain the heamodynamic parameters from real patient studies.

Figure 25. Exponential fitting on the right ventricle activity curve	Figure 26. Exponential fitting on the left left ventricle activity curve
Figure 27. Complete result image of a heamodynamic evaluation	Figure 28. The first pass process of the heart blood flow by image presentation

Let us do the following remark about the obtained results: the $$[T_{Max}; T_2]$ interval - i.e. between the first recircular point and the maximum activity point - can’t be appropriate and optimal for all cases of real measured curves as was presented above for exponential curve fitting to the descending branch of the theoretical first pass curve due to the higher and variable noise level. According to the real curve shape it is possible to make some changes that are supported by the clinical evaluation systems as well. Consequently, these modifications in interval selection are maintained for both curve fittings and integral value calculations by the processing systems. Nevertheless, the above figures show the appropriate conception of interval selection according to theoratical interval definition.

Another tool and method can be efficiently applicable in order to model and characterize the pure first pass process and calculate the “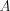” area to be presented in chapter 4.3.2 at Left-Right shunt determination. There the first pass process was characterized by gamma function
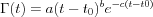$$\Gamma (t) = a(t-t_0)^b e^{-c(t-t0)}$ fitting. Let us consider figure 29. below, where the first pass process is presented by 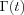$$\Gamma (t)$ function modelling.

Figure 29. Clean first pass process modelled by {EQUATION(size=

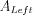The algorithms of heamodynamic parameters calculation are completely same as has been described above in case of exponential fitting method. The only differences are in “ $A_{Right}$ ” and “ $A_{Left}$ ” integral calculations due to the applied 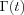$$\Gamma (t)$ function fitting.
In case of right ventricle: 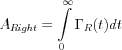$$A_{Right} = \int\limits_{0}^{\infty} \Gamma_R(t)dt$
In case of left ventricle: 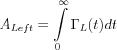$$A_{Left} = \int\limits_{0}^{\infty} \Gamma_L(t)dt$
where 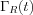$$\Gamma_R (t)$ is the fitted gamma function on 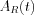$A_R(t) right ventricle time activity curve in $$[t_{R_\gamma 1};t_{R_\gamma 2}]$ interval.
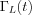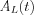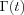$$\Gamma_L (t)$ has a similar meaning for the $A_L(t) left ventricle time activity curve in the $$[t_{L_{ \gamma }1};t_{L_{\gamma}2}]$ interval as well. Some of the concerns, examples and features of $$\Gamma (t)$ function fitting have been described and presented in chapter 4.3.2 through figures too.

Export

Medical Imaging

Site Language: English

Determination of heart’s heamodynamic parameters by first pass procedure

Sidebar

Medical Imaging

Site Language: English