ML-EM variations: MAP-EM,OSEM

Angolstruktura » Introduction to the mathematics of medical imaging » Algebraic Image Reconstruction » ML-EM variations: MAP-EM,OSEM

MAP-EM

The ML-EM method can be extended simply with Bayesian estimates adding a prior.
Now the previous section Eq.(1) can be extended with the condition 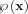$$ \wp \left ( \mathbf{x} \right )$ .
In the M step the derivative of 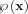$$ \wp \left ( \mathbf{x} \right )$ shows up, that finally becomes:
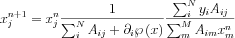
$$ x_{j}^{n+1}=x_{j}^{n}\frac{1}{\sum_{i}^{N}A_{ij}+\partial _{i }\wp\left ( x \right )}\frac{\sum_{i}^{N}y_{i}A_{ij}}{\sum_{m}^{M}A_{im}x_{m}^{n}}$
The method formed as shown is called the MAP-EM method

OSEM

A further and additional variation ito the ML-EM and MAP-EM methods is the Ordered Subset Expectation Maximization (OSEM). The basic idea is that the sums on i is only carried out for a subset of N. In emission tomography this could mean a transaxial grouping that the subsets homogeonously but sparsely fill the sinogram space.The subsets are chosen such that in the subsequent iterations the whole sinogram space is covered, so the subset changes iteration by iteration and their union gives the whole domain.

Outlook

In the algebraic reconstructionsection
we intended to show only the basic ideas of the currently used techniques. It is particularly true for transmission tomography where the ML-EM method is rarely used, but some alternatives are being tested like the gradient or the convex algorithm.

Export

Medical Imaging

Site Language: English