PMT (Photomultiplier Tube)
The Hungarian name of the PMT (‘fotoelektron-sokszorozó’, literally photoelectron multiplier) is more accurate.
The main parts of the PMT are the photocathode, the window and the dynodes.
Photocathode
It converts some of the incident photons into photoelectrons. We distinguish between the reflection mode cathode and the transmission mode cathode, applied in different cases. The photon-to-photoelectron conversion efficiency is very important: as we will see later, it is the factor which statistical noise depends on: 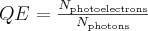
There are many types of photocathodes depending on the spectrum, the intensity, the size, etc. of the photon beam, e.g. CsI (blind over 200 nm), Cs-Te (sensitive to UV and visible light), Sc-Sn, etc. In the PMTs of the devices used in nuclear medicine bialkali photocathodes are the most widespread (Sb-Rb-Cs or Sb-K-Cs, approximately 300-650 nm, which fit well to the BGO, GSO, LSO, LYSO, NaI scintillators). The quantum efficiency of a photocathode is typically 20-30% (i.e. it converts about 20-30% of the incoming photons into electrons). Characteristically it exhibits low dark current. The thermal emission in the photocathode can be a significant source of the dark current of the PMT.
PMT window
The main types are UV, Quartz, MgF2 and borosilicalite (glass), according to the fields of application, temperature and price. The combination of a borosilicalite window and a bialkali photocathode is the most important for us.
Dynodes
The basic idea is the following: some sort of good conductor (nickel, stainless steel, copper-beryllium alloy) is covered with a coating out of which it is easy to knock out electrons and the work function is low (MgO, GaP, Ga-A-P).
The gain of the PMT
Let us have a look at Figure 1. The accelerated electrons arriving from the previous stage hit the dynode and knock out more low-energy (~eV) electrons. The multiplication is proportional to the energy of the incoming electrons, and so to the acceleration voltage. The acceleration voltage of each stage is proportional to the high voltage (HV), so the dependence of the gain on the number n of the stages:

It is typically in the order of 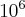 , so a PMT switched on without light block-out will get damaged immediately.
, so a PMT switched on without light block-out will get damaged immediately.
The electrons are the slowest before the first stage, they can get diverted by a magnetic field (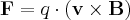 ). As a result of that and because of geometry the voltage step is usually larger before the first stage. Under the influence of a magnetic field the gain almost always decreases, so the optimization of the electron trajectories is not planned accordingly. A PMT rarely operates well over 100 Gauss, but this depends on the dynode arrangement. E.g. the detector head in SPECT is sensitive to the changes in the Earth’s magnetic field (relative to the direction of the PMTs) due to its rotation. That is why permalloy magnetic shielding around the PMTs is useful.
). As a result of that and because of geometry the voltage step is usually larger before the first stage. Under the influence of a magnetic field the gain almost always decreases, so the optimization of the electron trajectories is not planned accordingly. A PMT rarely operates well over 100 Gauss, but this depends on the dynode arrangement. E.g. the detector head in SPECT is sensitive to the changes in the Earth’s magnetic field (relative to the direction of the PMTs) due to its rotation. That is why permalloy magnetic shielding around the PMTs is useful.
Statistical noise and energy resolution of the PMT
If N scintillation photons are created during the scintillation process and each of them escape from the crystal with a probability p, then the probability that k photons escape follows the binomial distribution:
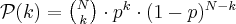
Typically there are a few thousand photons. The factorial of large numbers is unmanageable.
If we take the limit 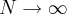 and
and 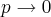 while
while 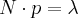 , and k are fixed, then
, and k are fixed, then
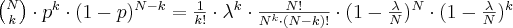
Taking the limit, we get the Poisson distribution with parameter 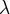 :
:
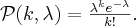
Therefore, the Poisson distribution can be used as a good approximation of the binomial distribution. Let us not forget that according to the definition 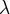 is the number of the photons that will expectedly escape,
is the number of the photons that will expectedly escape, 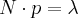 . The expected value of such a random variable is
. The expected value of such a random variable is 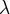 , its variance is also
, its variance is also 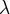 , thus its relative standard deviation is
, thus its relative standard deviation is 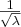 , the inverse of the square root of the escaping photons. If the light yield is four times as high, the relative standard deviation of the number of photons is twice as low.
, the inverse of the square root of the escaping photons. If the light yield is four times as high, the relative standard deviation of the number of photons is twice as low.
These are the photons out of which photoelectrons are created in the PMT. The photoelectrons produced during the light pulses caused by a gamma photon arriving at the detector generate an electrical signal. We wish to figure out the energy of the gamma photon absorbed by the scintillator by examining the amplitude of the signal.
What is the statistical uncertainty of this? The process of the escaping photons generating photoelectrons once again follows a Poisson distribution. We will not prove the fact that the cascades of Poisson processes yield another Poisson process.
If  , the Gaussian distribution can be used as a good approximation of the Poisson distribution (see Figure 4).
, the Gaussian distribution can be used as a good approximation of the Poisson distribution (see Figure 4).
This further approximation proves to be useful because it is known that the variance and the full width at half maximum of a Gaussian distribution are proportional to each other, namely
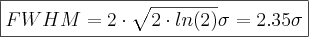
This way the full width at half maximum of the distribution, which plays an important role in the energy resolution, can be estimated immediately.
Out of the 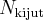 photons that get to the photocathode from the crystal only
photons that get to the photocathode from the crystal only 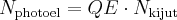 become photoelectrons due to the efficiency of the photocathode. This many photoelectrons generate a signal based on which the relative resolution of the detector (in practice usually given in percentage as the ratio of the full width at half maximum to the expected value so that the result does not depend on the units of measurement):
become photoelectrons due to the efficiency of the photocathode. This many photoelectrons generate a signal based on which the relative resolution of the detector (in practice usually given in percentage as the ratio of the full width at half maximum to the expected value so that the result does not depend on the units of measurement):
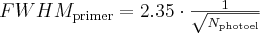
This is the standard deviation of the so-called primary electrons appearing on the photocathode. We cannot expect a better energy resolution than that because the PMT cannot increase the amount of information. In 1938 Shockley and Pierce proved that an electron multiplier with n stages (after the photocathode the PMT can be considered an electron multiplier), each stage has an amplification R, will yield a relative full width at half maximum (a resolution) of
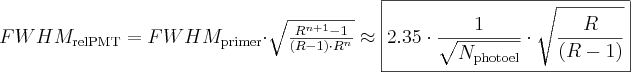
at the last stage.
In the last inequality we used the fact that 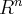 , the gain, is a large number and if we subtract one, it practically does not change. Therefore, this is the lowest statistical estimate of the energy resolution of the PMT when a fixed number of photons are generated (=in case of gamma radiation with a fixed energy), thus this is the full width at half maximum of the statistical uncertainty of the PMT signal.
, the gain, is a large number and if we subtract one, it practically does not change. Therefore, this is the lowest statistical estimate of the energy resolution of the PMT when a fixed number of photons are generated (=in case of gamma radiation with a fixed energy), thus this is the full width at half maximum of the statistical uncertainty of the PMT signal.
In a PMT with 10 stages and a gain of 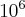 , which is considered a typical value,
, which is considered a typical value, 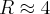 so the 10 stages of the PMT multiplies the relative standard deviation of the incoming photon statistics by
so the 10 stages of the PMT multiplies the relative standard deviation of the incoming photon statistics by 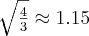 only.
only.
We can claim that the PMT is a very pure amplifier. We can see now why quantum efficiency is important: it is the factor on which the number of photoelectrons depends. In practice this is a low estimate, the true energy resolution of the PMT can be twice as bad due to thermal noise (creation of photoelectrons on the photocathode, on the dynodes), HV noise, the inhomogeneity of the scintillation crystal, etc. However, this model does not apply to semiconductor detectors, since the processes occurring in them cannot be correctly approximated by the Poisson distribution. The Fano factor is the ratio of the relative standard deviation predicted by the Poisson statistics to the observed relative standard deviation. In some cases the real value is better than the value suggested by the Poisson distribution, e.g. the Fano factor of a semiconductor silicon detector is 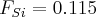 , of a germanium detector it is
, of a germanium detector it is 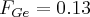
In case of positioning based on the Anger principle the point of entry of the gamma photon is estimated using the amplitude of the PMT signals, so the relative standard deviation plays an important role in positioning as well.
PSPMT (Position Sensitive PMT)
PSPMTs consist of many small PMTs in a common housing. They are expensive but they allow for good positioning, in small animal PET devices they are practically exclusively used. It would be pointless to apply them in human PET scanners because of non-collinearity.