Phase Encoding

Book for Physicists » The principles of MRI » Development of spatial resolution » Phase Encoding

During the readout, a gradient can only be applied along a straight line. (This line does not need to be one of the distinguished directions of the coordinate system. If our device can generate gradients in three perpendicular directions, using their linear combinations a gradient can be created in an arbitrary direction.) An option to image the three-dimensional space is to repeat the excitation and the measurements many times, while the direction of the gradient is rotated. If we stay in two dimensions, we obtain a series of linear projections: data of the same sort as are obtained in the X-ray CT. In that case, the two-dimensional image can be reconstructed with the aid of the inverse Radon transform. Yet, this technique is rarely used in practice, since a method called phase encoding leads to a set of data which can be reconstructed more easily.
Notice that the fact that the gradient was turned on during the readout was not made use of in formulae (13), (14) and (16) (15 ?). If we manage to collect k values sufficiently densely with arbitrarily timed gradients, then the effective spin density can be reconstructed. Our “natural strategy” is to continuously collect the measurement points with the gradient turned on during the echo, but we can also carry out more experiments, set a specific k value with the gradient before each echo, and then collect a sole measurement point during the echo. Of course, this second method is rarely used instead of the first one, all the more together with it. If the data are collected along a given direction in a way that the experiment is repeated, a certain phase distribution is set with the gradients in every step, and then a sole point is read out, we talk about phase encoding.
If more gradients are applied, the general form of the local field is the following:

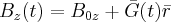$B_{z}(t)=B_{0z}+\bar{G}(t)\bar{r}$ (17 ?16?)

The following formula is obtained for the phase:

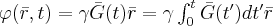$\varphi(\bar{r},t)=\gamma\bar{G}(t)\bar{r}=\gamma\intop_{0}^{t}\bar{G}(t')dt'\bar{r}$ (18 ?17?)

Thus, the quantity introduced in formula (14) will be a vector:

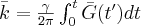$\bar{k}=\frac{\gamma}{2\pi}\intop_{0}^{t}\bar{G}(t')dt$ (19 ?18?)

The relationship between the spin density and the measured signal is expressed by a three-dimensional Fourier integral:

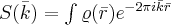$S(\bar{k})=\int\varrho(\bar{r})e^{-2\pi i\bar{k}\bar{r}}$ (20 ?19?)

How should all this be understood? By applying the gradients we move in the abstract 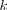$k$ space. The measurement points fixed one after the other in time can be interpreted as the signals measured in the different points of the 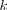$k$ space. It is stated that if the 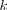$k$ space is bejár ? sufficiently densely, then the spin density can be reconstructed with the aid of the Fourier transform.

Export

Medical Imaging

Site Language: English

Phase Encoding

Sidebar

Medical Imaging

Site Language: English