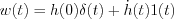Loading...
 
PDF Print

Relation between the step response function and weighting function of linear shift invariant system

Let’s it try to find relation between the step response functions and the weighting functions (i.e. impulse response function) of the linear shift invariant systems.
It is known from the previous chapters, if the weighting function of a linear shift invariant system is 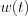$ w(t) and the input function is 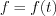$ f=f(t) general function, then the output response is:

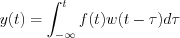
$ y(t)=\int_{-\infty}^tf(t)w(t-\tau)d\tau

It is known also, if the input function is the Heavyside unit step function, then the output response is the step response function as well:

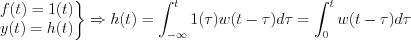
$ \left.\begin{matrix} f(t)=1(t)\\ y(t)=h(t) \end{matrix}\right\} \Rightarrow h(t)=\int_{-\infty}^t1(\tau)w(t-\tau)d\tau=\int_0^tw(t-\tau)d\tau

Apply the following substitute in order to execute the integral:
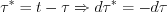
$ \tau^*=t-\tau\Rightarrow d\tau^*=-d\tau
The new integral bounds are:
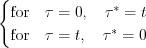
$ \begin{cases} \text{for} \quad \tau=0, \quad \tau^*=t \\\text{for} \quad \tau=t, \quad \tau^*=0 \end{cases}

Consequently, 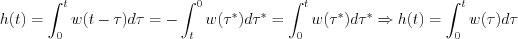$ h(t) =\int_0^tw(t-\tau)d\tau =-\int_t^0w(\tau^*)d\tau^*=\int_0^tw(\tau^*)d\tau^* \Rightarrow h(t)= \int_0^tw(\tau)d\tau

Let’s it apply the inverse operation of the integral, i.e. apply the weak derivative:
$ w(t)= \frac{\delta h(t) }{\delta t}= \frac{\delta \left [ 1(t)h(t) \right ] }{\delta t}=h(0)\delta(t) +1(t) \frac{dh(t) }{dt}
$ w(t)=h(0)\delta(t)+ \dot{h}(t)1(t)

Export

Medical Imaging

Site Language: English

Log in as…