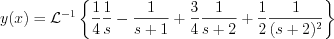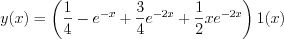PDF Print

S6.


Solution:

Let’s execute the Laplace transformation both side of the equation!

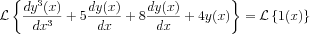
$ \mathcal{L}\left\{\frac{dy^{3}(x)}{dx^{3}}+5\frac{dy(x)}{dx}+8\frac{dy(x)}{dx}+4y(x)\right\} = \mathcal{L}\left\{1(x)\right\}

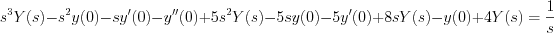
$ s^{3}Y(s)-s^{2}y(0)-sy'(0)-y''(0)+5s^{2}Y(s)-5sy(0)-5y'(0)+8sY(s)-y(0)+4Y(s)=\frac{1}{s}

Let’s substitute the available initial conditions 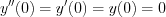$ y''(0)=y'(0)=y(0)=0

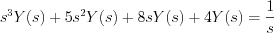
$ s^{3}Y(s)+5s^{2}Y(s)+8sY(s)+4Y(s)=\frac{1}{s}

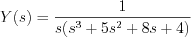
$Y(s)=\frac{1}{s(s^{3}+5s^{2}+8s+4)}

Result of the inverse Laplace transformation will give 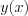$y(x), as the general solution:

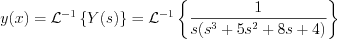
$ y(x)=\mathcal{L}^{-1}\left\{Y(s)\right\} = \mathcal{L}^{-1}\left\{\frac{1}{s(s^{3}+5s^{2}+8s+4)}\right\}

First step is to find the roots of denominator:

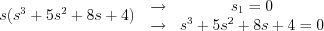
$ s(s^{3}+5s^{2}+8s+4)\begin{matrix} \quad \rightarrow & s_{1}=0\\ \quad \rightarrow & s^{3}+5s^{2}+8s+4=0 \end{matrix}

One real root of the third order polynomial is 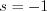$s=-1, which means the polynomial can be expressed by fully-factored form as follow
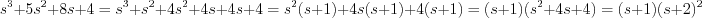
$s^{3}+5s^{2}+8s+4=s^{3}+s^{2}+4s^{2}+4s+4s+4 = s^{2}(s+1)+4s(s+1)+4(s+1)=(s+1)(s^{2}+4s+4)=(s+1)(s+2)^{2}

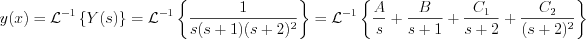
$y(x)=\mathcal{L}^{-1}\left\{Y(s)\right\}=\mathcal{L}^{-1}\left\{\frac{1}{s(s+1)(s+2)^{2}}\right\} = \mathcal{L}^{-1}\left\{\frac{A}{s}+\frac{B}{s+1}+\frac{C_{1}}{s+2}+\frac{C_{2}}{(s+2)^{2}}\right\}

Next task is to determine the coefficients of the partial function
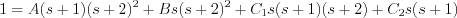
$1=A(s+1)(s+2)^{2}+Bs(s+2)^{2}+C_{1}s(s+1)(s+2)+C_{2}s(s+1)
Solution of this equation will give the coefficients.

Let’s see the following cases:
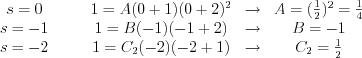
$ \begin{matrix} s=0 & \quad & 1=A(0+1)(0+2)^{2} & \rightarrow & A=(\frac{1}{2})^{2}=\frac{1}{4}\\ s=-1 & \quad & 1=B(-1)(-1+2) & \rightarrow & B=-1\\ s=-2 & \quad & 1=C_{2}(-2)(-2+1) & \rightarrow & C_{2}=\frac{1}{2} \end{matrix}

If 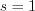$s=1 will be substituted, then 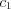$c_{1} can be obtained.

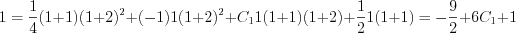
$1=\frac{1}{4}(1+1)(1+2)^{2}+(-1)1(1+2)^{2}+C_{1}1(1+1)(1+2)+\frac{1}{2}1(1+1)=-\frac{9}{2}+6C_{1}+1

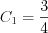
$C_{1}=\frac{3}{4}

Consequently, the solution is $y(x)=\mathcal{L}^{-1}\left\{\frac{1}{4}\frac{1}{s}-\frac{1}{s+1}+\frac{3}{4}\frac{1}{s+2}+\frac{1}{2}\frac{1}{(s+2)^{2}}\right\}

$y(x) = \left(\frac{1}{4}-e^{-x}+\frac{3}{4}e^{-2x}+\frac{1}{2}xe^{-2x}\right)1(x)

 
(Return to the Problems)

Export

Medical Imaging

Site Language: English

Log in as…