Basics of 2D imaging

The concept of 2D imaging is quite similar to the one-dimensional sequences: we use gradient fields to move in the 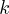-space of the sample plane and sometimes 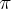pulses to mirror the 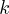-vector of the system.

We usually categorize the 2D imaging sequences based on the number of 90° excitations they use to cover the whole plane. This way we define one-shot sequences that acquire the whole 2D image with one excitation and multi-shot sequences that use multiple excitation pulses.

In clinical practice multi-shot imaging sequences usually acquire one 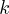-line per excitation. The typical sequence that does not use refocusing pulses is shown in Figure 1. As usual in sequence diagrams this figure shows only one cycle of the sequence which is repeated until the whole 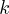-plane is measured. In each cycle we move to the far left side of the plane with the negative 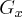gradient to a position along 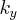determined by the actual value of 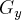in the cycle. After that we measure one line along .

Figure 1. Left: One cycle of 2D imaging sequence. The ladder with the arrow in Gy represents the alternation of this gradient in each cycle. Right: trajectory in k-space.

 

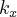The direction of our movement in 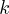-space during the acquisition is called "readout" or "frequency encoding" direction, and the corresponding gradient is called "readout gradient". In the literature this direction is usually denoted by 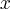, however the gradient is often abbreviated as 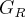(with R denoting Readout). The other direction in which the movement is not measured is referred to as "phase encoding" direction and usually denoted by 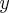. The corresponding gradient is denoted by 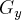or 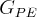where PE abbreviates Phase Encoding.

Of course this sequence can be modified by using refocusing pulses. This is usually done to reduce the effect of 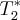decay during the measurement of one line. Such a spin echo sequence is shown in Figure 2. As can be seen this time we go to the positive side of the 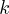-plane and mirror our position by the 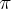pulse followed by the acquisition of a single line.

Figure 2. Left: One cycle of 2D spin echo imaging sequence. The ladder with the arrow in Gy represents the alternation of this gradient in each cycle. Right: trajectory in k-space.

 

A whole 2D image can be achieved much faster by a single-shot sequence. This type of sequences uses only one 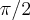excitation to acquire the image. The fundamental sequence of this type is the so-called Echo Planar Imaging or EPI. The sequence diagram of EPI is shown in Figure 3.

Figure 3. Left: Echo Planar Imaging (EPI) sequence. Right: trajectory in k-space.

 
We note here that there are numerous 2D imaging sequences that differ mostly in the way of covering the 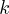-space and in the usage of refocusing 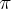pulses.