Classical Description

In case of a macroscopic sample the dynamics of the moments can also be described classically. (In this case a “macroscopic sample” is much smaller than the relevant voxel sizes of imaging.) First of all, it can be stated that the gyromagnetic ratio defined in formula (1) remains unchanged. Magnetization 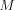$M$ can be introduced as the sum of magnetic moments in a given volume. In an external field a torque is exerted on the magnetization:

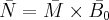$\bar{N}=\bar{M}\times\bar{B_{0}}$ (5)

It is also known that the torque is equal to the change in angular momentum:

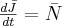$\frac{d\bar{J}}{dt}=\bar{N}$ (6)

By making the two formulae equivalent and eliminating the angular momentum, an equation of motion of the magnetization is obtained:

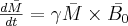$\frac{d\bar{M}}{dt}=\gamma\bar{M}\times\bar{B_{0}}$ (7)

Let us examine the resulting motion. If the magnetization and the external field are not parallel to each other, then the change in magnetization will be perpendicular both to the external field and to the magnetization itself, i.e. the magnetization vector moves along the lateral surface of a cone, the axis of which points in the direction of the external field, and it precesses about the magnetic field 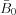$\bar{B}_{0}$ at angular velocity 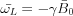$\bar{\omega_{L}}=-\gamma\bar{B}_{0}$ . This is called Larmor precession.
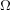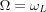In order to make the description simpler, let us change to another coordinate system, the z axis of which is the same as that of the system used so far (laboratory coordinate system), and which rotates about it at angular velocity $\Omega$ . If $\Omega=\omega_{L}$ , then the magnetization does not move in the rotating system. 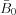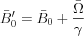The inertial forces emerging in the rotating system formally act like an axial magnetic field with a magnitude of $\frac{\Omega}{\gamma}$ , thus the form of the equation of motion does not change; $\bar{B}_{0}$ just has to be substituted by an effective magnetic field $\bar{B}_{0}'=\bar{B}_{0}+\frac{\bar{\Omega}}{\gamma}$ .
The aforementioned statements about relaxation can also be integrated into the equation of motion.

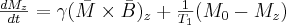$\frac{dM_{z}}{dt} & = & \gamma(\bar{M}\times\bar{B})_{z}+\frac{1}{T_{1}}(M_{0}-M_{z})$ (8)

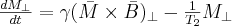
$\frac{dM_{\bot}}{dt} & = & \gamma(\bar{M}\times\bar{B})_{\bot}-\frac{1}{T_{2}}M_{\bot}$

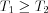where is the equilibrium magnetization. In the formula above an individual relaxation time can be presumed for the relaxation of the transversal component. It is obvious that only cases in which $T_{1}\geq T_{2}$ can occur, since by the time the equilibrium magnetization is built up, the transversal component has to vanish. However, the transversal components may vanish faster. This means that the coherence of the precessing atomic moments is lost. They keep precessing about the external field, but since they get out of phase, their resultant vanishes. This process is called spin-spin relaxation. In magnetic resonance studies an orthogonal time-dependent magnetic field 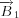$\overrightarrow{B}_{1}$ with a small amplitude is applied for the system besides the static field 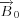$\overrightarrow{B}_{0}$ , which displaces the magnetization from its equilibrium. The magnetic field 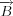$\overrightarrow{B}$ is the resultant of these two fields. Let the angular frequency of the microwave field be $\omega$ , and let it be circularly polarized, i.e. 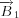let $\overrightarrow{B}_{1}$ rotate in the (x,y) plane. Let us write formulae (8) in a rotating coordinate system. Let the axial effective field be denoted by 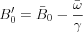$B_{0}'=\bar{B}_{0}-\frac{\bar{\omega}}{\gamma}$ , and let the transversal field 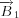$\overrightarrow{B}_{1}$ be parallel to the x axis of the rotating system. Thus formulae (8) take on the following form:

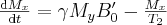
$\frac{\textrm{d}M_{x}}{\textrm{d}t} & = & \gamma M_{y}B_{0}'-\frac{M_{x}}{T_{2}}$

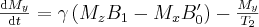$\frac{\textrm{d}M_{y}}{\textrm{d}t} & = & \gamma\left(M_{z}B_{1}-M_{x}B_{0}'\right)-\frac{M_{y}}{T_{2}}$ (9)

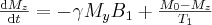
$\frac{\textrm{d}M_{z}}{\textrm{d}t} & = & -\gamma M_{y}B_{1}+\frac{M_{0}-M_{z}}{T_{1}}$

If the angular frequency of the excitation (the angular velocity of the coordinate system) is equal to the Larmor frequency, then the external field 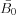$\bar{B_{0}}$ vanishes completely in the rotating system and the magnetization will precess about the exciting field 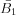$\bar{B_{1}}$ Therefore, the magnetization can be rotated by an arbitrary angle ( 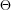$\Theta$ ) by a pulse with an appropriate magnitude ( 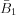$\bar{B}_{1}$ ) and duration ( $\tau$ ):

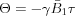
$\Theta=-\gamma\bar{B}_{1}\tau$

The “sheet music”, the “sequence” of the simplest MR experiment is depicted in Figure 1.

Figure 1. The “sequence” of the simplest MR experiment. Time is depicted horizontally, while the intensity of the appropriate quantity corresponding to the specific lines is depicted vertically. Here an exponentially vanishing oscillating signal, which occurs after a 90° radio pulse, can be observed. This signal is called free induction decay (FID).

The original document is available at http://549552.cz968.group/tiki-index.php?page=Classical+Description