Doppler Ultrasound

The frequency of the reflected wave is higher (lower) than that of the emitted wave, if the reflecting surface is moving towards (away from) the observer.

Let us assume that the wavefront of a wave moving with velocity c at time t=0 has just reached the reflecting surface which moves with velocity v, and the entire wave leaves this surface at time 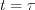$t=\tau$ . The wavefront has covered distance 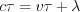$c\tau=v\tau+\lambda$ (from the initial position of the reflecting surface) in time $\tau$ (dotted wave). Thus, 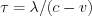$\tau=\lambda/(c-v)$ . 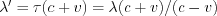The initial point of the reflected wave has covered the same distance from the initial position of the reflecting surface, but in the meantime the reflecting surface has moved with $v\tau$ ; therefore, the wavelength of the reflected wave is $\lambda'=\tau(c+v)=\lambda(c+v)/(c-v)$ . 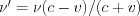Based on this formula, the frequency of the reflected wave can be calculated: $\nu'=\nu(c-v)/(c+v)$ .

Using the difference between the frequencies the velocity of the reflecting surface can be easily calculated:

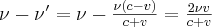
$\nu-\nu'=\nu-\frac{\nu(c-v)}{c+v}=\frac{2\nu v}{c+v}$

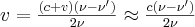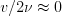and hence $v=\frac{(c+v)(\nu-\nu')}{2\nu}\approx \frac{c(\nu-\nu')}{2\nu}$ , because $v/2\nu \approx 0$ .

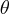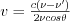If the velocities c and v enclose an angle $\theta$ , then the formula to be used is the following: $v=\frac{c(\nu-\nu')}{2\nu cos\theta}$ .

The device emits a rustling sound, the intensity of which is proportional to the measured velocity v; this helps the determination of the position and the direction of the veins, so that the direction of the blood flow and the emitted ultrasound are parallel to each other, and the device also displays the velocity of the flowing blood numerically.
The direction and the velocity of the flow is usually displayed with a colourful scale on the grey cross-sectional image.

Normal

Reduced

The examination of venous circulation

The original document is available at http://549552.cz968.group/tiki-index.php?page=Doppler+Ultrasound