Repeated pulses and contrast

In this section we investigate the effect of repeating the pulses, meaning that in the pulse starting time the magnetization does not equal to its equilibrium value but it is the result of the previous sequences. First we deal with FID. We measure the time from the first 90° pulse applied to the equilibrium magnetization. The pulse repetition time is denoted by 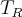$T_R$ . For the first pulse, $t<T_R$ :

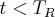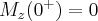$\label{repeated_FID1} M_z (0^+) = 0$ (1)

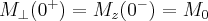$\label{repeated_FID2} M_{\bot}(0^+) = M_z (0^-) = M_0$ (2)

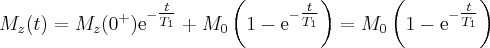$\label{repeated_FID3} M_z (t) = M_z (0^+) \mathrm{e}^{- \tfrac{t}{T_1}} + M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t}{T_1}} \right ) = M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t}{T_1}} \right)$ (3)

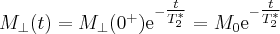$\label{repeated_FID4} M_{\bot} (t) = M_{\bot}(0^+) \mathrm{e}^{-\tfrac{t}{T^*_2}} = M_0 \mathrm{e}^{-\tfrac{t}{T^*_2}}$ (4)

and for the second pulse, 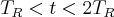$T_R < t < 2T_R$ :

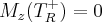$\label{repeated_FID5} M_z (T_R^+) = 0$ (5)

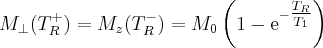$\label{repeated_FID6} M_{\bot}(T_R^+) = M_z (T_R^-) = M_0 \left( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right)$ (6)

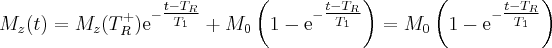$\label{repeated_FID7} M_z (t) = M_z (T_R^+) \mathrm{e}^{- \tfrac{t-T_R}{T_1}} + M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t-T_R}{T_1}} \right ) = M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t-T_R}{T_1}} \right)$ (7)

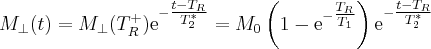$\label{repeated_FID8} M_{\bot} (t) = M_{\bot}(T_R^+) \mathrm{e}^{-\tfrac{t-T_R}{T^*_2}} = M_0 \left( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right ) \mathrm{e}^{-\tfrac{t-T_R}{T^*_2}}$ (8)

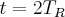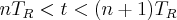If we substitute $t=2T_R$ into equations (7) and (8) we found that the dynamics will be the same for all the subsequent pulses, that is, for $nT_R < t < (n+1)T_R$ :

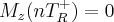$\label{repeated_FID9} M_z (nT_R^+) = 0$ (9)

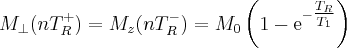$\label{repeated_FID10} M_{\bot}(nT_R^+) = M_z (nT_R^-) = M_0 \left( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right)$ (10)

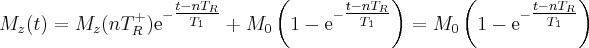$\label{repeated_FID11} M_z (t) = M_z (nT_R^+) \mathrm{e}^{- \tfrac{t-nT_R}{T_1}} + M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t-nT_R}{T_1}} \right ) = M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t-nT_R}{T_1}} \right)$ (11)

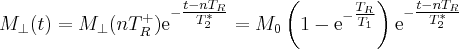$\label{repeated_FID12} M_{\bot} (t) = M_{\bot}(nT_R^+) \mathrm{e}^{-\tfrac{t-nT_R}{T^*_2}} = M_0 \left( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right ) \mathrm{e}^{-\tfrac{t-nT_R}{T^*_2}}$ (12)

From these we can see that in the case of a repeated FID sequence the signal will always be enveloped by the 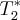$T^*_2$ decay. However, this can be modulated with 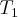$T_1$ relaxation with an appropriate repetition time: if 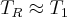$T_R \approx T_1$ then the exponential term in (12) will modify the signal depending on the longitudinal relaxation, while with the condition $T_R \gg T_1$ the effect of 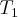$T_1$ decay can be eliminated from the signal.

The situation is a bit more exciting in the case of spin echo. We measure time from the first 90° pulse as usual, and denote the time between the 90° and the 180° pulses with 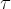$\tau$ . For the first sequence:

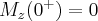$\label{repeated_SE1} M_z (0^+) = 0$ (13)

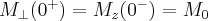$\label{repeated_SE2} M_{\bot}(0^+) = M_z (0^-) = M_0$ (14)

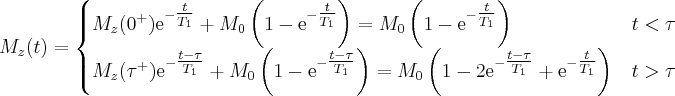$\label{repeated_SE3} M_z(t)= \begin{cases} M_z (0^+) \mathrm{e}^{- \tfrac{t}{T_1}} + M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t}{T_1}} \right ) = M_0 \left ( 1 - \mathrm{e}^{- \tfrac{t}{T_1}} \right) & t < \tau \\ M_z(\tau^+)\mathrm{e}^{-\tfrac{t-\tau}{T_1}} + M_0 \left( 1 - \mathrm{e}^{-\tfrac{t-\tau}{T_1}} \right) = M_0 \left ( 1 - 2 \mathrm{e}^{-\tfrac{t-\tau}{T_1}} + \mathrm{e}^{-\tfrac{t}{T_1}} \right) & t > \tau \end{cases}$ (15)

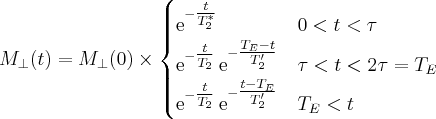$\label{repeated_SE4} M_{\bot} (t) = M_{\bot} (0) \times \begin{cases} \mathrm{e}^{ - \tfrac{t}{T^*_2} } & 0 < t < \tau \\ \mathrm{e}^{ - \tfrac{t}{T_2} } \hspace{2pt} \mathrm{e}^{ - \tfrac{T_E - t}{T'_2} } & \tau < t < 2 \tau = T_E \\ \mathrm{e}^{ - \tfrac{t}{T_2} } \hspace{2pt} \mathrm{e}^{ - \tfrac{t - T_E}{T'_2} } & T_E < t \end{cases}$ (16)

For the second sequence, i. e. for 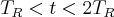$T_R < t < 2T_R$ :

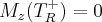$\label{repeated_SE5} M_z (T_R^+) = 0$ (17)

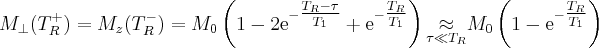$\label{repeated_SE6} M_{\bot}(T_R^+) = M_z (T_R^-) = M_0 \left ( 1 - 2 \mathrm{e}^{-\tfrac{T_R-\tau}{T_1}} + \mathrm{e}^{-\tfrac{T_R}{T_1}} \right ) {\underset{\tau \ll T_R}{\approx}} M_0 \left ( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right )$ (18)

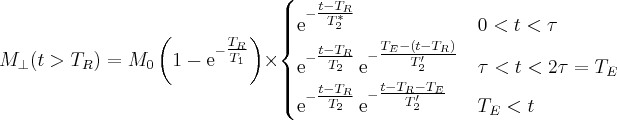$\label{repeated_SE7} M_{\bot} (t>T_R) = M_0 \left ( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right ) \times \begin{cases} \mathrm{e}^{ - \tfrac{t-T_R}{T^*_2} } & 0 < t < \tau \\ \mathrm{e}^{ - \tfrac{t-T_R}{T_2} } \hspace{2pt} \mathrm{e}^{ - \tfrac{T_E - (t-T_R)}{T'_2} } & \tau < t < 2 \tau = T_E \\ \mathrm{e}^{ - \tfrac{t-T_R}{T_2} } \hspace{2pt} \mathrm{e}^{ - \tfrac{t-T_R - T_E}{T'_2} } & T_E < t \end{cases}$ (19)

From these the accurate expression for the echo maximum after several pulses:

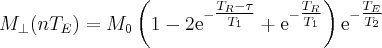$\label{repeated_SE8} M_{\bot}(nT_E) = M_0 \left ( 1 - 2 \mathrm{e}^{-\tfrac{T_R-\tau}{T_1}} + \mathrm{e}^{-\tfrac{T_R}{T_1}} \right ) \mathrm{e}^{-\tfrac{T_E}{T_2}}$ (20)

If we assume the half echo time $\tau$ to be much smallet than the repetition time 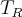$T_R$ :

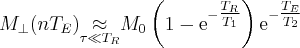$\label{repeated_SE9} M_{\bot}(nT_E) {\underset{\tau \ll T_R}{\approx}} M_0 \left ( 1 - \mathrm{e}^{-\tfrac{T_R}{T_1}} \right ) \mathrm{e}^{-\tfrac{T_E}{T_2}}$ (21)

From equation (21) several conclusions can be drawn.

1) If we set the repetition time to be large compared to the longitudinal relaxation and the echo time to be small, meaning $T_R \gg T_1$ and $T_E \ll T_2$ then the exponential terms vanish or become approx. equal to one, and the signal will be proportional simply to the eqilibrium value of the magnetization. In this way we can eliminate the effect of relataxion from the signal and observe only the spatial proton density. This method is called "proton density weighting (PD)".

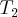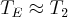2) If the repetition time remans large but the echo time is in the same order of magnitude as the $T_2$ relaxation, i. e. $T_R \gg T_1$ and $T_E \approx T_2$ then the effect of longitudinal relaxation will still be eliminated but the 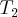$T_2$ relaxation will alternate the signal as the exponential term containing this will not vanish. This is called "T2 weighting"} in imaging processes.

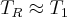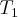3) If the echo times is small and the repetition time is approximately the same as the longitudinal relaxation, that is, if $T_R \approx T_1$ and $T_E \ll T_2$ then the effect of transverse relaxation is eliminated but the $T_1$ relaxation modifies the signal. This is called "T1 weighting".

We note here that the aboving ideas have some practical limitations: the repetition time of course can be as long as we want but the reduction of 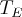$T_E$ is limited by technical reasons. Therefore the condition $T_E \ll T_2$ is sometimes hard to achieve, especially if the transverse relaxation is fast.

The original document is available at http://549552.cz968.group/tiki-index.php?page=Repeated+pulses+and+contrast