Measurable Signal

The quantity which can directly be measured is the time derivative of the transversal magnetization (in the laboratory coordinate system). Using formula (8), it takes on the following form:

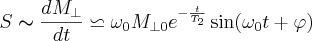$S\thicksim\frac{dM_{\bot}}{dt}\backsimeq\omega_{0}M_{\bot0}e^{-\frac{t}{T_{2}}}\sin(\omega_{0}t+\varphi)$ (10)

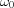where $\omega_{0}$ is the Larmor frequency.
If the phase, or the magnitude of the magnetization is not homogeneous, then the signal is generated as the resultant of the local signals. (In case the phase can be time-dependent, the frequency can always be considered constant.)
During measurements usually coils are used, which can be modelled in a simplified way as two perpendicular loops along the x and y axes. With the aid of these not only the magnitude of the rotating magnetization can be measured, but also its phase, which can be expressed as a complex number. Using this formalism and permitting inhomogeneities as well, the signal will be:

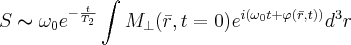$S\thicksim\omega_{0}e^{-\frac{t}{T_{2}}}\int M_{\bot}(\bar{r},t=0)e^{i(\omega_{0}t+\varphi(\bar{r},t))}d^{3}r$ (11)

The high-frequency oscillation can be transformed easily both mathematically and in the measuring electronics. Let us write the factors in the proportionality that have not been denoted so far, and the initial transversal magnetization into one coefficient, let us name it effective spin density and denote it by $\varrho$ This contains factors such as the sensitivity of the measuring coils and the electronics, the magnetization of the sample and the resonance frequency. If we ignore the relaxation, the signal can be written in the following form:

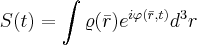$S(t)=\int\varrho(\bar{r})e^{i\varphi(\bar{r},t)}d^{3}r$ (12)

Export

Medical Imaging

Site Language: English

Measurable Signal

Sidebar

Medical Imaging

Site Language: English