Precession-quantum mechanical description
In quantum mechanics both angular and magnetic moment are represented by hermitic operators characterized by their eigenvalues and eigenstates. For the square of the angular momentum, 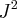 the possible eigenvalues are
the possible eigenvalues are
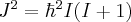 (1)
(1)
Where I is the integer or half-integer quantum number of the angular momentum. For the projection of the angular momentum to a specific directon, for example to 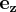 :
:
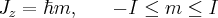 (2)
(2)
For a single spin-half particle, there is a similar relation between angular and magnetic moment as it was in the classical case, that is
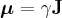 (3)
(3)
Therefore the matrix elements of a specific component of the magnetic moment can be computed using the angular momentum operator. With the bra-ket notation introduced by Dirac, the matrix element with the states described by quantum numbers (I, m) and (I, m'):
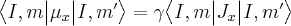 (4)
(4)
If the particle is placed into external magnetic field, its originally degenerated spectrum splits based on the projection of the magnetic moment to the external field - this is called the Zeeman effect. The descriptive Hamiltonian:
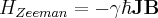 (5)
(5)
If the external magnetic fields is $B_0$ then the splitted energy levels are
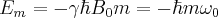 (6)
(6)
In general, the wavefunction of the particle is a sum of the eigenstates with different weights, each multiplied by the time propagation term with one of the energies described above
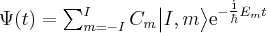 (7)
(7)
Using equations (4) and (7) we can compute the mean value of 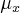 for a spin-half particle, exploiting the fact that in this case
for a spin-half particle, exploiting the fact that in this case 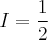 .
.
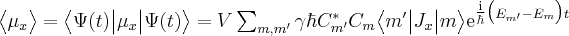 (8)
(8)
Now we express the 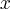 component of the angular momentum with the usual ladder operators
component of the angular momentum with the usual ladder operators
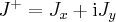 (9)
(9)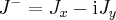 (10)
(10)
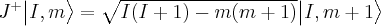 (11)
(11)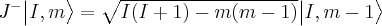 (12)
(12)
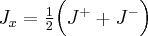 (13)
(13)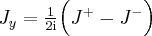 (14)
(14)
After that substitute (6), and (11)-(14) in (8), consider the fact that both 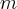 and
and 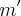 can only take the values of
can only take the values of  and
and 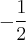 , and that eigenstates with different
, and that eigenstates with different 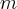 values are orthogonal to each other:
values are orthogonal to each other:
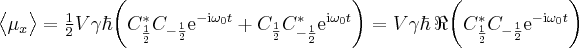 (15)
(15)
Without loss of generality we can assume that 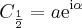 and
and 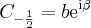 for some
for some 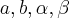 real numbers. Furthermore, the normalization criterion requires that
real numbers. Furthermore, the normalization criterion requires that 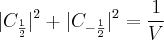 , which allowes us to write
, which allowes us to write 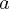 and
and 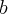 as
as 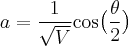 and
and 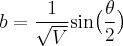 . Using these expressions (15) becomes the following:
. Using these expressions (15) becomes the following:
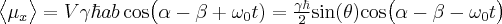 (16)
(16)
In an absolutely similar manner one can show that the mean values of the other two components of magnetic moment will be the following:
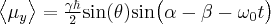 (17)
(17)
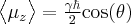 (18)
(18)
With these expressions we have presented that the quantum mechanical calculations of a spin-half particle also shows the precession movement in the sense of mean values as equations (16), (17) and (18) describe a vector that precesses around axis  with angular frequency
with angular frequency 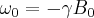 .
.