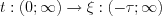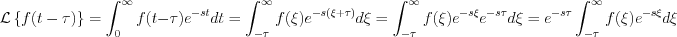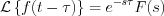Properties and rules of Laplace transformation

1.) Laplace transformation of addition operation can be executed by element due to the linear property of Laplace transformation,

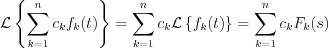
$$ \mathcal{L}\left\{\sum_{k=1}^{n}c_k f_k (t) \right\} = \sum_{k=1}^{n}c_k \mathcal{L}\left\{f_k (t)\right\} = \sum_{k=1}^{n}c_k F_k (s)$

2.) Laplace transformation of derivative

Let 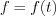$ f=f(t) function a general step function, where its Laplace transformation is 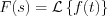$$ F(s)=\mathcal{L} \left \{ f(t) \right \}$ .The question is: How is possible to derive the Laplace transformation of 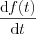$$ \frac{\mathrm{d}f(t)}{ \mathrm{d}t}$ derived function.

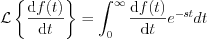$$ \mathcal{L} \left \{ \frac{\mathrm{d}f(t)}{ \mathrm{d}t} \right \} = \int_0^{\infty}\frac{\mathrm{d}f(t)}{ \mathrm{d}t}e^{-st}dt$ where the improper integral has to carry out.

Let’s use the partial integral rule:
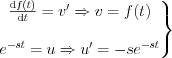$$ \left.\begin{matrix} \frac{\mathrm{d} f(t)}{\mathrm{d} t}={v}'\Rightarrow v=f(t) \\ \\ e^{-st}=u\Rightarrow {u}'=-se^{-st} \end{matrix}\right\}$ , where ’ denotes the derivative by „t”.

Consequently,
$$ \mathcal{L} \left \{ \frac{\mathrm{d}f(t)}{ \mathrm{d}t} \right \} = \int_0^{\infty}\frac{\mathrm{d}f(t)}{ \mathrm{d}t}e^{-st}dt= \left [ f(t)e^{-st} \right ]_0^{\infty} - \int_0^{\infty}(-se^{-st})f(t)dt$

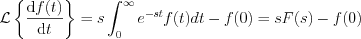
$$ \mathcal{L} \left \{ \frac{\mathrm{d}f(t)}{ \mathrm{d}t} \right \} = s\int_0^{\infty}e^{-st}f(t)dt-f(0)=sF(s)-f(0)$

Then apply the rule by inductive way for higher (i.e. “n” order) derivative, by using the conditions, that 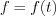$ f=f(t) general step function, and “n” ordered continuously differentiable in the 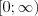$$ \left [ 0;\infty \right )$ interval.

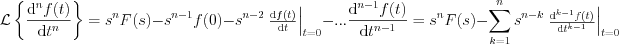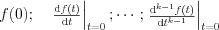$$ \mathcal{L} \left \{ \frac{\mathrm{d}^nf(t)}{ \mathrm{d}t^n} \right \} =s^nF(s)-s^{n-1}f(0)-s^{n-2}\left.\begin{matrix} \frac{\mathrm{d} f(t)}{\mathrm{d} t} \end{matrix}\right|_{t=0}-...\frac{\mathrm{d}^{n-1} f(t)}{\mathrm{d} t^{n-1}}=s^nF(s)-\sum_{k=1}^ns^{n-k}}}\left.\begin{matrix} \frac{\mathrm{d}^{k-1} f(t)}{\mathrm{d} t^{k-1}} \end{matrix}\right|_{t=0}$ , where $$ f(0); \quad \left.\begin{matrix} \frac{\mathrm{d}f(t)}{\mathrm{d} t} \end{matrix}\right|_{t=0} ;\cdots; \left.\begin{matrix} \frac{\mathrm{d}^{k-1} f(t)}{\mathrm{d} t^{k-1}} \end{matrix}\right|_{t=0}$ are the higher (max. “n-1” order) ordered derivative value at 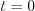$t=0 point, i.e. at the entry point /these are the initial conditions/.

3.) Laplace transformation of integrals

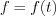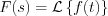Let $ f=f(t) be a general step function and $$ F(s)=\mathcal{L} \left \{ f(t) \right \}$ .

Let’s determine the following Laplace transformation 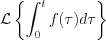$$ \mathcal{L} \left \{\int_0^t f(\tau)d\tau \right \}$ .
Definition of the Laplace transformation will be used as previously:
$$ \mathcal{L} \left \{\int_0^t f(\tau)d\tau \right \} = \int_0^{\infty} \left [ \int_0^t f(\tau)d\tau \right ] e^{-st}dt$

Let’s use the partial integral rule as happened above at the derivative case:

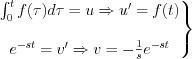
$$ \left.\begin{matrix} \int_0^t f(\tau)d\tau=u \Rightarrow {u}'=f(t)\\ \\ e^{-st}={v}'\Rightarrow v=-\frac{1}{s}e^{-st} \end{matrix}\right\}$

$$ \mathcal{L} \left \{\int_0^t f(\tau)d\tau \right \} = \int_0^{\infty} \left [ \int_0^t f(\tau)d\tau \right ] e^{-st}dt=\left [-\frac{1}{s}e^{-st} \int_0^t f(\tau)d\tau \right ]_0^{\infty} - \int_0^{\infty} \left ( -\frac{1}{s} \right ) e^{-st}f(t)dt$

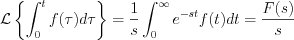
$$ \mathcal{L} \left \{\int_0^t f(\tau)d\tau \right \} =\frac{1}{s} \int_0^{\infty} e^{-st}f(t)dt=\frac{F(s)}{s}$

Similar to the “n” order derivative determination can be driven the Laplace transformation of “n” ordered integral.
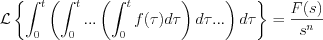
$$ \mathcal{L} \left \{ \int_0^t \left (\int_0^t \text{...} \left (\int_0^tf(\tau)d\tau \right ) d\tau \text{...} \right )d\tau \right \} =\frac{F(s)}{s^n}$

4.) Theorem of similar

Let 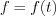$ f=f(t) be a general step function and 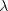$$ \lambda$ an optional real number.

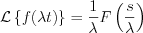
$$ \mathcal{L} \left \{ f(\lambda t)\right \} =\frac{1}{\lambda}F \left ( \frac{s}{\lambda} \right )$

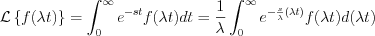
$$ \mathcal{L} \left \{ f(\lambda t)\right \} = \int_0^{\infty} e^{-st}f(\lambda t)dt=\frac{1}{\lambda} \int_0^{\infty} e^{-\frac{s}{\lambda}(\lambda t)}f(\lambda t)d(\lambda t)$

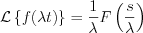
$$ \mathcal{L} \left \{ f(\lambda t)\right \} =\frac{1}{\lambda}F\left (\frac{s}{\lambda} \right )$

This property of Laplace transformation is according to the property of linearity (see above the linear properties of Fourier and Laplace transformation).

5.) Attenuation theorem

Let 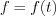$ f=f(t) be a general step function and 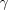$$ \gamma$ an optional real number.

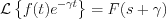
$$ \mathcal{L} \left \{ f(t)e^{-\gamma t}\right \} =F(s+\gamma)$

It is known, if 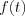$ f(t) general step function, then 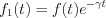$$ f_1(t)=f(t) e^{-\gamma t}$ is the same.

Consequently, 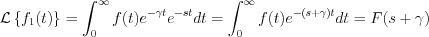$$ \mathcal{L} \left \{ f_1(t) \right \} =\int_0^{\infty} f(t)e^{-\gamma t} e^{-st}dt=\int_0^{\infty} f(t)e^{-(s+\gamma) t}dt=F(s+\gamma)$

6.) Shift theorem

Figure 27. and 28. show the graphs of general step function and shifted general step function.

Figure 27.

Figure 28.

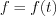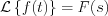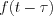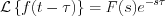
If Laplace transformation of $ f=f(t) is $$ \mathcal{L} \left \{ f(t) \right \} = F(s)$ , then Laplace transformation of $$ f(t-\tau)$ is: $$ \mathcal{L} \left \{ f(t-\tau) \right \} = F(s) e^{-s\tau}$

In order to proof the shift theorem, apply the original definition of Laplace transformation:
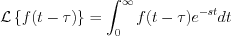
$$ \mathcal{L} \left \{ f(t-\tau) \right \} = \int_0^{\infty}f(t-\tau)e^{-st}dt$
Apply the following new variable: 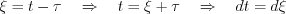$$ \xi=t-\tau \quad \Rightarrow \quad t=\xi+\tau \\ \quad \Rightarrow \quad dt=d\xi$
Consequently the new integral bounds are: $$ t:(0;\infty) \rightarrow \xi:(-\tau;\infty)$

$$ \mathcal{L} \left \{ f(t-\tau) \right \} = \int_0^{\infty}f(t-\tau)e^{-st}dt=\int_{-\tau}^{\infty}f(\xi)e^{-s(\xi+\tau)}d\xi =\int_{-\tau}^{\infty}f(\xi)e^{-s\xi}e^{-s\tau}d\xi= e^{-s\tau}\int_{-\tau}^{\infty}f(\xi)e^{-s\xi}d\xi$

$$ \mathcal{L} \left \{ f(t-\tau) \right \}= e^{-s\tau}F(s)$

Export

Medical Imaging

Site Language: English

Properties and rules of Laplace transformation

Sidebar

Medical Imaging

Site Language: English