Signal detection

Book for Physicists » Magnetic Resonance Imaging » Theoretical background of magnetic resonance » Signal detection

In the previous sections we have focused on the effects of external fields to magnetic moments and the time evolution of the magnetization. Now we proceed to the question how can this precessing magnetization be measured. The answer is, of course, induction. The fundamental idea is to rotate the spins from their equilibrium direction to the transverse plane with an applied RF pulse and measure the voltage induced by the precessing magnetization in the coil around the sample. In the followings the detailed deduction of the induced signal is presented.

From the integral form of the 3rd Maxwell equation we know that a time-varying magnetic flux induces electromotive force (emf or simply voltage) in a coil:

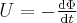$\label{induction} U = - \frac{ \mathrm{d} \Phi} {\mathrm{d} t}$ (1)

The magnetic flux can be computed with the integration of magnetic field on a surface, but sometimes it is more convenient to use the Stokes theorem to calculate the flux by the integration of the vector potential on a closed line bounding the desired surface.

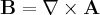$\label{B_rot_A} \mathbf{B} = \nabla \times \mathbf{A}$ (2)

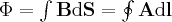$\label{flux_with_vector_potential} \Phi = \int \mathbf{B} \mathrm{d} \mathbf{S} = \oint \mathbf{A} \mathrm{d} \mathbf{l}$ (3)

When dealing with conventional electric currents, the vector potential can be calculated with the current density:

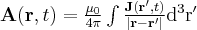$\label{vector_potential} \mathbf{A} ( \mathbf{r} , t ) = \frac{\mu_0}{4 \pi} \int \frac {\mathbf{J} ( \mathbf{r'} , t)} {| \mathbf{r} - \mathbf{r'} |} \mathrm{d^3r'}$ (4)

But we do not have real electric currents, only magnetization. However, from the differential form of the 4th Maxwell equation and the definition of the magnetizing field we can construct a current density associated with magnetization.

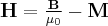$\label{H_definition} \mathbf{H} = \frac{ \mathbf{B}} {\mu_0} - \mathbf{M}$ (5)

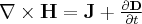$\label{maxwell_4} \nabla \times \mathbf{H} = \mathbf{J} + \frac{\partial \mathbf{D}}{\partial t}$ (6)

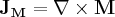$\label{H_definition} \mathbf{J_M} = \nabla \times \mathbf{M}$ (7)

Substituting this to (3) and (4) we get an expression to magnetic flux containing the magnetization:

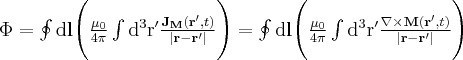$\label{flux_with_vector_potential2} \Phi = \oint \mathrm{d} \mathbf{l} \Bigg ( \frac{\mu_0}{4 \pi} \int \mathrm{d^3r'} \frac {\mathbf{J_M} ( \mathbf{r'} , t )} {| \mathbf{r} - \mathbf{r'} |} \Bigg ) = \oint \mathrm{d} \mathbf{l} \Bigg ( \frac{\mu_0}{4 \pi} \int \mathrm{d^3r'} \frac {\nabla \times \mathbf{M} ( \mathbf{r'} , t )} { | \mathbf{r} - \mathbf{r'} |} \Bigg )$ (8)

Using integration by parts and leaving the surface terms as there are no magnetization in the infinitely far point:

$\label{flux_with_vector_potential3} \Phi = \frac{\mu_0}{4 \pi} \int \mathrm{d^3r'} \oint \mathrm{d} \mathbf{l} \Bigg [ \bigg ( - \nabla' \frac{1}{ | \mathbf{r} - \mathbf{r'} | } \bigg ) \times \mathbf{M} (\mathbf{r'} , t ) \Bigg ]$ (9)

We can now use the vector identity 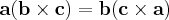$\mathbf{a} (\mathbf{b} \times \mathbf{c} ) = \mathbf{b} ( \mathbf{c} \times \mathbf{a} )$ and get:

$\label{flux_with_vector_potential4} \Phi = \int \mathrm{d^3r'} \mathbf{M} (\mathbf{r'} , t ) \underbrace{ \Bigg [ \nabla \times \oint \frac{\mu_0}{4 \pi} \frac{\mathrm{d} \mathbf{l}}{ | \mathbf{r} - \mathbf{r'} | } \Bigg ] }_{\mathcal{B}^{rec}}$ (10)

If we compare the term marked as 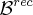$\mathcal{B}^{rec}$ in (10) with (2) and (4) it becomes clear that this 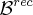$\mathcal{B}^{rec}$ tells us the effectiveness of the coil at the point $\mathbf{r}$ , i. e. the magnetic field generated at the point $\mathbf{r}$ with a unit current flowing in the coil. Because of the invariance of Maxwell's equations to a time reversal transformation, this coil efficiency also characterizes the receiving sensitivity, i. e. 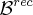if there is a time-varying magnetic moment at point {EQUATION}$\mathbf{r}${EQUATION}, it induces an electromotive force in the coil proportional to $\mathcal{B}^{rec}$ . This statement, also referred to as the "principle of reciprocity", we will not justify in this section; a detailed explanation can be found in the literature.

Now we have our expression for the magnetic flux with the receiving sensitivity 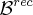$\mathcal{B}^{rec}$ . The signal we measure both in NMR and MRI is the voltage induced by the precessing magnetization in the coil(s) around the sample or the patient. Therefore our signal 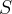$S$ will be proportional to the time derivative of the magnetic flux in (10) (the proportionality factor usually differs from 1 because of the measurement electronics):

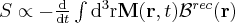$\label{NMR_signal} S \propto - \frac{ \mathrm{d} } { \mathrm{d} t } \int \mathrm{d^3r} \mathbf{M} (\mathbf{r} , t ) \mathcal{B}^{rec} (\mathbf{r} )$ (11)

The magnetization contains two types of time dependence: the Larmor precession and the exponential decays. The frequencies associated with these terms are in the order of magnitude of 10-100 $\mathrm{Hz}$ and 100 $\mathrm{MHz}$ , respectively. Therefore the time derivative of the exponential decays are negligible beside the Larmor precession, so one can say that only the precession gives contribution to the signal. It implies that we can only measure the transversal components of the magnetization, or in the complex formalism, $M_+$ . 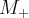As a reminder the time and space dependence of this is:

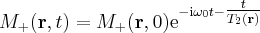$M_+ (\mathbf{r}, t) = M_+ (\mathbf{r}, 0) \mathrm{e}^{ - \mathrm{i} \omega_0 t - \tfrac {t}{T_2 ( \mathbf{r} )}}$ (12)

So our signal will be:

$\label{NMR_signal2} S \propto \omega_0 \int \mathrm{d^3r} \hspace{3pt} \mathrm{e}^{ - \tfrac{t}{T_2 (\mathbf{r})} } \bigg [ \mathcal{B}^{rec}_x (\mathbf{r} ) \Re \Big ( \mathrm{i} M_+ ( \mathrm{r} , t ) \mathrm{e}^{ - \mathrm{i} \omega_0 t } \Big ) + \mathcal{B}^{rec}_y (\mathbf{r} ) \Im \Big ( \mathrm{i} M_+ ( \mathrm{r} , t ) \mathrm{e}^{ - \mathrm{i} \omega_0 t } \Big ) \bigg ]$ (13)

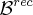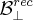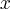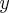For the complex description to work we also define the transversal component of $\mathcal{B}^{rec}$ denoted by $\mathcal{B}^{rec}_{\bot}$ , and write up the $x$ and $y$ components with this:

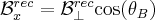$\label{B_rec_transverse} \mathcal{B}^{rec}_x = \mathcal{B}^{rec}_{\bot} \mathrm{cos} (\theta_B)$ (14)

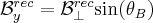$\mathcal{B}^{rec}_y = \mathcal{B}^{rec}_{\bot} \mathrm{sin} (\theta_B)$ (15)

\end{subequations}

Using this method the signal will be the following:

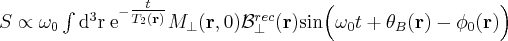$\label{NMR_signal3a} S \propto \omega_0 \int \mathrm{d^3r} \hspace{3pt} \mathrm{e}^{ - \tfrac{t}{T_2 (\mathbf{r})} } M_{\bot} (\mathbf{r} , 0 ) \mathcal{B}^{rec}_{\bot} (\mathbf{r} ) \mathrm{sin} \Big ( \omega_0 t + \theta_B (\mathbf{r} ) - \phi_0 (\mathbf{r} ) \Big )$ (16)

In the spatially independent limit, i. e. when everything in (16) is independent from $\mathbf{r}$ the signal will be as follows:

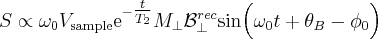$\label{NMR signal_spatially_independent} S \propto \omega_0 V_{\mathrm{sample}} \mathrm{e}^{ - \tfrac{t}{T_2} } M_{\bot} \mathcal{B}^{rec}_{\bot} \mathrm{sin} \Big ( \omega_0 t + \theta_B - \phi_0 \Big )$ (17)

Export

Medical Imaging

Site Language: English

Signal detection

Sidebar

Medical Imaging

Site Language: English