Analysis of linear systems in extended frequency space

The description of linear system will be extended into the complex frequency domain 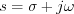$$s=\sigma + j\omega$ in the following chapters, in order to investigate what kind of association may be created between the various system responses.

Definition: Let 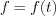$f=f(t) real variable and real as well as complex value general step function:

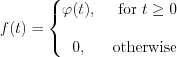$$ f(t)=\left\{\begin{matrix} \varphi (t), &\text{ for } t \geq 0\\ \\ 0, & \text{ otherwise } \end{matrix}\right.$ , as well as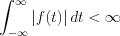 $$\int_{-\infty}^{\infty}\left|f(t)\right| dt < \infty$

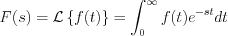$$F(s)=\mathcal{L}\left\{f(t)\right\} = \int_{0}^{\infty}f(t)e^{-st}dt$ operation is the Laplace transformation of $f(t) . Sufficient condition of Laplace transformation is the $f(t) function should be absolutely integrable (the condition is not necessary, but sufficient).

Laplace transformation is linear, i.e.:

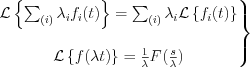$$ \left.\begin{matrix} \mathcal{L}\left\{\sum_{(i)}\lambda_i f_i (t) \right\} = \sum_{(i)}\lambda_i \mathcal{L} \left\{f_i (t)\right\} \\ \\ \mathcal{L} \left\{ f(\lambda t)\right\} = \frac{1}{\lambda} F(\frac{s}{\lambda}) \end{matrix}\right\}$ , where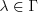 $$\lambda \in \Gamma$

Let’s see, the relation between the Laplace and Fourier transformation. It is known from the above mentioned, the sufficient condition of both Laplace and Fourier transformation is 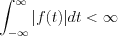$$ \int_{-\infty}^{\infty}|f(t)| dt < \infty$ , i.e. 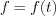$f=f(t) should be absolutely integrable.

Fourier transformation: 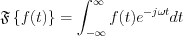$$\mathfrak{F}\left\{f(t)\right\} = \int_{-\infty}^{\infty}f(t)e^{-j\omega t}dt$

Laplace transformation: 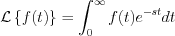$$\mathcal{L}\left\{f(t)\right\} = \int_{0}^{\infty}f(t)e^{-st}dt$

Apply the following substitution in the Laplace transformation expression: 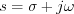$$s=\sigma +j\omega$ .
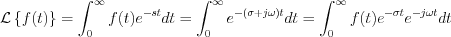
$$ \mathcal{L}\left\{f(t)\right\} = \int_{0}^{\infty}f(t)e^{-st}dt = \int_{0}^{\infty}e^{-(\sigma +j\omega)t}dt = \int_{0}^{\infty}f(t)e^{-\sigma t}e^{-j\omega t}dt$

It is known from the definition of Laplace transformation, that 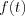$f(t) should be general step function. If 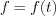$f=f(t) is general step function, then 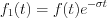$$f_1 (t) = f(t)e^{-\sigma t}$ is general step function too.

Consequently
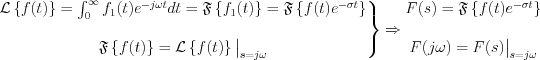
$$ \left.\begin{matrix} \mathcal{L}\left\{f(t)\right\} = \int_{0}^{\infty}f_1 (t)e^{-j\omega t}dt = \mathfrak{F}\left\{f_1 (t)\right\} = \mathfrak{F}\left\{f(t)e^{-\sigma t}\right\}\\ \\ \mathfrak{F}\left\{f(t)\right\} = \mathcal{L}\left\{f(t)\right\} \big{|}_{s=j\omega} \end{matrix}\right\} \Rightarrow \begin{matrix} F(s)=\mathfrak{F}\left\{f(t)e^{-\sigma t}\right\} \\ \\ F(j\omega) = F(s) \big{|}_{s=j\omega} \end{matrix}$

The conclusions are the followings:
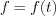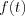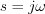Under the conditions, that $f=f(t) is general step function and absolutely integrable, the Laplace transformation of $f(t) among $$s=j\omega$ complex axis (i.e. among the imaginary axis) gives the Fourier transformation. Consequently, it is possible to see, the domain of Fourier transformation is the 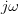$$j\omega$ imaginary axis, while the domain of Laplace transformation is the complete complex plane. Another approach is existing also, that the Laplace transformation of 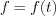$f=f(t) is possible to derive from the Fourier transformation of 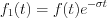$$f_1 (t) = f(t)e^{-\sigma t}$ function. Consequently 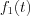can be expressed by the inverse Fourier transformation as follow:

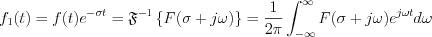
$$ f_1 (t) = f(t)e^{-\sigma t} = \mathfrak{F}^{-1}\left\{F(\sigma+j\omega )\right\} = \frac{1}{2\pi} \int_{-\infty}^{\infty}F(\sigma+j\omega )e^{j\omega t}d\omega$

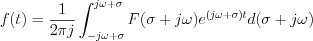
$$ f(t) = \frac{1}{2\pi j}\int_{-j\omega+\sigma}^{j\omega+\sigma}F(\sigma+j\omega)e^{(j\omega+\sigma)t}d(\sigma+j\omega)$

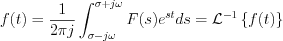$$ f(t) = \frac{1}{2\pi j}\int_{\sigma-j\omega}^{\sigma+j\omega}F(s)e^{st}ds = \mathcal{L}^{-1}\left\{f(t)\right\}$ where the expression of inverse Laplace transformation is obtained,
and called Rieman-Melling integral.

The original document is available at http://549552.cz968.group/tiki-index.php?page=Analysis+of+linear+systems+in+extended+frequency+space